-
PDF
- Split View
-
Views
-
Cite
Cite
Florian Karpfinger, Tobias M. Müller, Boris Gurevich, Green's functions and radiation patterns in poroelastic solids revisited, Geophysical Journal International, Volume 178, Issue 1, July 2009, Pages 327–337, https://doi.org/10.1111/j.1365-246X.2009.04116.x
Close - Share Icon Share
Summary
A consistent and unified formulation of Green's functions for wave propagation in poroelastic solids based on Biot's theory is given. Over the last decades various authors have made the attempt to derive different Green's tensor representation for poroelastic solids. Due to the various possible combinations of field variables and source terms the different solutions differ significantly. The main solutions are reviewed and compared. It is shown that these previously reported representations of Green's tensors can be used in a complementary sense such that all possible combinations of field variables and source types are included. As a new element we introduce the concept of moment tensors for poroelasticity. This allows us to describe sources in poroelastic solids in a consistent manner. With the help of the moment tensor concept a pressure source acting on the fluid phase is introduced as well as dipole sources and double-couple sources. To visualize the results, radiation patterns for all the discussed sources are constructed. The shape of the radiation patterns of the fast compressional and shear wave is the same as in elastodynamics, however, the radiation characteristics of Biot's slow wave are superimposed. The relative magnitudes of the field variables shown in the radiation patterns can be very different for different source types. In particular, for any source acting in the fluid phase the pressure field is dominated by the Biot slow wave having compressional wave polarization.
1 Introduction
The equations for wave propagation in a fully fluid-saturated porous medium were first proposed by Biot 50 years ago Biot (1956a, b, 1962). Finding solutions of the two coupled poroelastic partial differential equations (PDE) in the presence of body force source terms is an important problem of wave mechanics in porous continua. In particular, if the source term is assumed to be a Dirac's delta function in time and space the solutions are referred to as Green's functions.
Green's functions have been obtained, under various assumptions, by various authors over the last decades. However, the Green's function differ since several combinations of field variables and source terms are possible in a poroelastic continuum. One set of field variables to represent the two PDEs are the solid displacement u and the relative solid-fluid displacement w. Another representation is based on u and the fluid displacement U. The third representation is a combination of a vector and a scalar variable, namely the solid displacement u and the pore-fluid pressure p. Each of these sets of field variables allows different source terms to be introduced. It is therefore not surprising that these different representations lead to a number of publications on Green's function in poroelasticity which are apparently different.
The first authors who derived Green's function for the dynamic poroelastic problem were Burridge & Vargas (1979). Their solution is based on the representation of the poroelastic wave equations using the solid displacement u and the relative solid-fluid displacement w as field variables. Burridge & Vargas (1979) applied the method of potential decomposition in conjunction with the Laplace transform. Explicit solutions were given for the far-field using the saddle point method. As only one type of source, which is a single force vector, is acting on the bulk phase but none on the fluid phase this solution solves the problem only partly. Using the Fourier transform method Norris (1985) derived Green's function in terms of the field variables (u, U). In addition to a source acting on the bulk phase a source vector in the fluid phase was added. In this work no explicit expression of Green's functions including all source types were given. Using a similar approach, Green's functions for poroelasticity were derived by Philippacopoulos (1998) and Lu et al. (2008). Norris (1994) used a different approach based on integration over a closed surface to derive Green's functions for poroelasticity.
Another way to determine Green's function for the equations of poroelasticity is to exploit the analogy with the PDEs of thermoelasticity (Kupradze 1979). This is possible since the PDEs of thermoelasticity and poroelasticity are mathematically equivalent (Norris 1992). The PDEs of poroelasticity can be represented in terms of one vectorial and one scalar field (u, p) (Bonnet 1987). Using the method of Kupradze, the frequency-domain Green's functions were derived by Bonnet (1987) and Boutin (1991). The source term acting on the fluid phase is a volume source V while the source in the bulk composite is a vector force F. In addition to the 3-D space Green's function the solution for the 2-D Green's function was also presented. Bonnet (1987) and Boutin (1991) pointed out that a source vector acting on the fluid phase (as used in Norris 1985) is unphysical.
Green's functions for coupled problem of elastic and electromagnetic wave propagation in porous media were presented by Pride & Haartsen (1996). These solutions can be reduced to the poroelastic Green's functions by neglecting the electromagnetic coupling terms (Müller & Gurevich 2005). On the basis of the field variables (u, w) representation, these Green's functions are given in the frequency space domain and are analogous to those given by Norris (1985). The advantage of the solution presented by Pride & Haartsen (1996) is that Green's functions were presented in the most explicit form separated in far-field and near-field contributions.
All Green's functions described above are presented in the frequency domain. In the presence of the Darcy dissipation term (Biot 1956a, b) it is not possible to analytically transform the results back into time domain without any further assumptions. A closed-form time domain solution for the case without viscous dissipation was given by Norris (1985). Two authors obtained Green's functions in time domain including the viscous dissipation term. Chen (1994a, b) gives solutions for 2-D and 3-D space using the Laplace transform method, however introducing approximations in the Laplace domain. Another approach is presented by Schanz & Pryl (2004) also using inverse Laplace transform method.
The solutions discussed here can be used to implement point sources in a number of seismic forward modelling algorithms in porous media, such as reflectivity (Allard et al. 1986), finite differences (Wenzlau & Müller 2009). The analytical solutions obtained in the paper can be used to benchmark the results of these algorithms. Furthermore, even when the source is physically located in a non-porous region of the medium, such as a fluid-filled borehole, explicit modelling of such geometrical configuration may require a prohibitively small grid size. This problem can be overcome by approximating the borehole as a series of point sources (Kurkjian et al. 1994). This approach requires the computation of the point source response. Thus, the poroelastic point source solutions presented in this paper can also help model the effect of a borehole in a poroelastic formation (including the effect of flow between the reservoir and the borehole).
In this paper, we provide a consistent formulation of Green's functions in poroelastic solids. The similarities and differences between the approaches described above are highlighted. In Section 2, we present the equations of linear poroelasticity. We provide a comparative review of Green's function of poroelasticity in Section 3. Radiation patterns for various source types are illustrated in Section 4. The concept of moment tensors is introduced to describe consistent source mechanisms in poroelastic solids including fluid and solid dipole sources as well as double-couple sources. Their radiation characteristics are also illustrated with the help of radiation patterns.
2 Equations of Poroelasticity





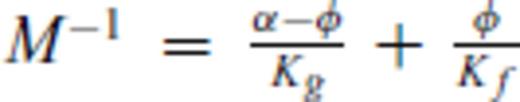 is the so-called pore space modulus and the saturated P-wave modulus is defined by H = Ksat+ 4/3μ. Ksat is the bulk modulus of the saturated medium that is related to the bulk moduli of the fluid Kf, solid Kg, and dry skeleton K by the Gassmann (1951) equation
is the so-called pore space modulus and the saturated P-wave modulus is defined by H = Ksat+ 4/3μ. Ksat is the bulk modulus of the saturated medium that is related to the bulk moduli of the fluid Kf, solid Kg, and dry skeleton K by the Gassmann (1951) equation 
Eqs (1)–(6) constitute the framework of wave propagation in fluid-saturated porous solids. To obtain Green's functions, appropriate source terms have to be introduced in eqs (1) and (2).
3 Green's Functions in Poroelasticity
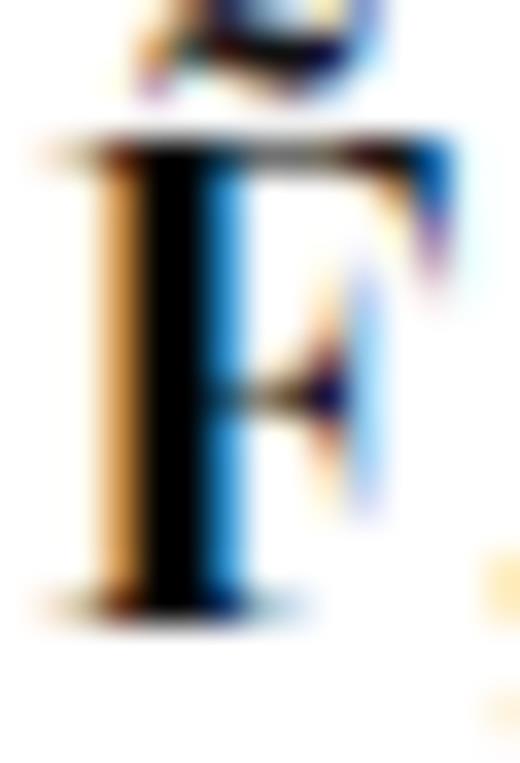 , and Green's function
, and Green's function 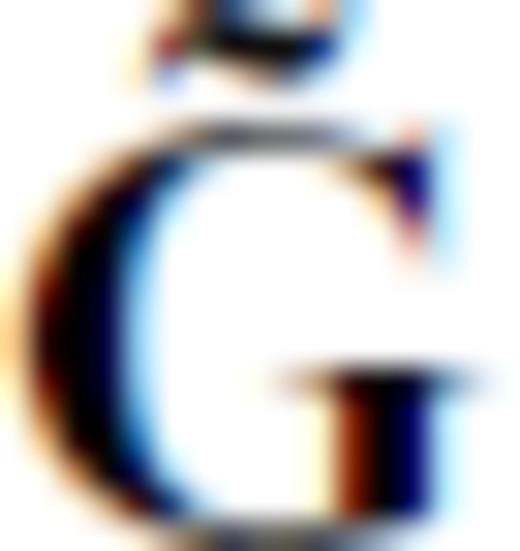 in time domain (Aki & Richards 2002) in the form of a convolution
in time domain (Aki & Richards 2002) in the form of a convolution 

A similar approach can be used to derive Green's function for poroelasticity. Eqs (1), (2), (4) and (5) can be represented as a pair of coupled wave equations by inserting the constitutive relations (4 and 5) into the equations of motion (1 and 2). However, depending on which of the three field variables (u, w or p) is eliminated, the set of wave equations consists of either six or four wave equations. Choosing u and w as field variables, one obtains two vector wave equations, whereas choosing u and p one obtains one vector and one scalar wave equation. Introducing source terms into these sets of equations allows to derive Green's functions that are different for both sets of equations. These two different Green's functions are reproduced in the next section based on the work of Pride & Haartsen (1996) and Boutin (1987).
3.1 Solid and relative fluid-solid displacement representation


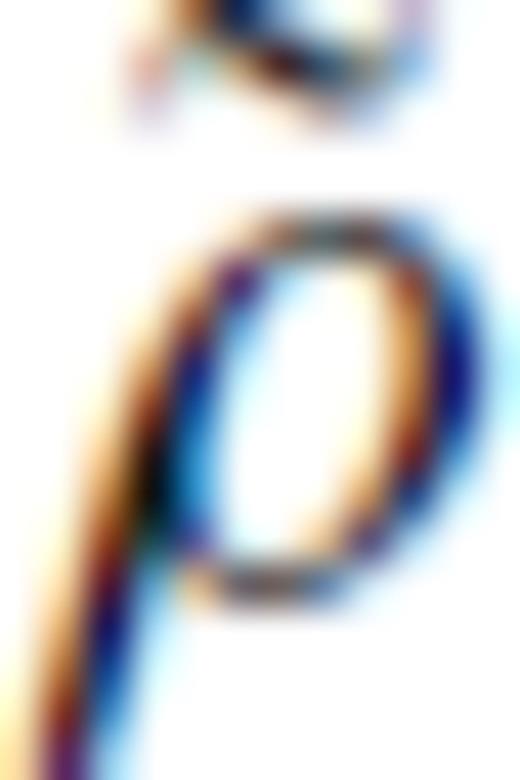 is
is 




 , longitudinal
, longitudinal 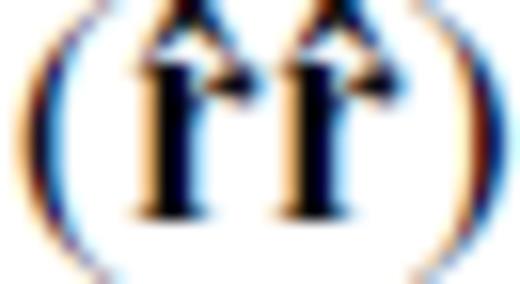 and near-field
and near-field 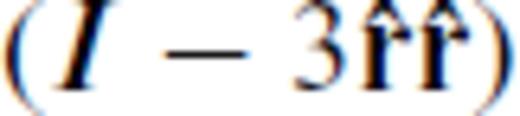 polarizations;
polarizations; 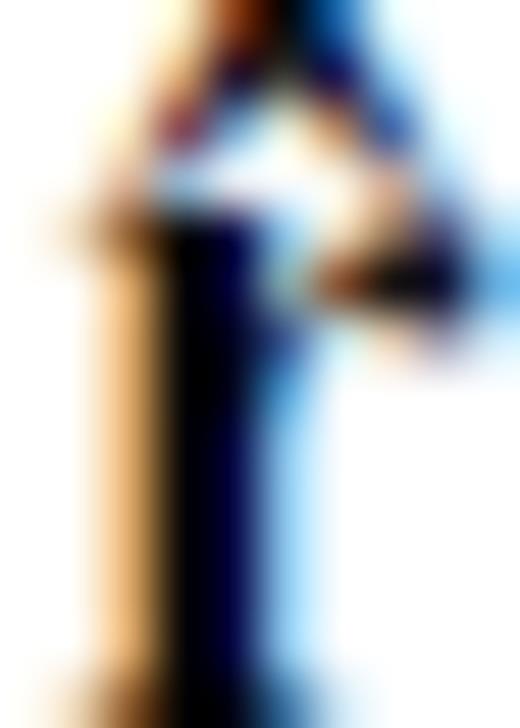 is the unit direction vector pointing from the source to the observation point.
is the unit direction vector pointing from the source to the observation point. 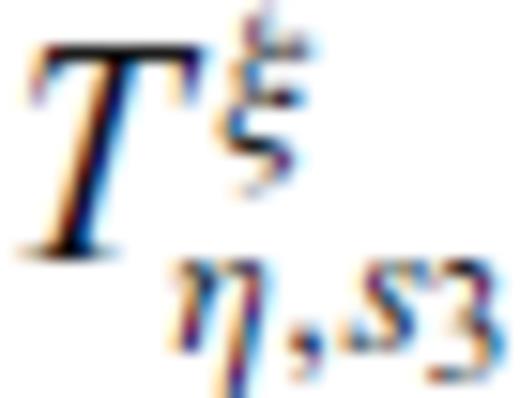 and
and  are the amplitudes of the transverse and longitudinal components. They are specified through the type of source indicated by ξ = {F, f} and the type of field indicated by η = {u, w} and the slownesses of the fast and slow compressional waves, s1, s2, and the shear wave, s3. Explicit expression of these three wave slownesses is given in Appendix A. The expressions for the amplitudes
are the amplitudes of the transverse and longitudinal components. They are specified through the type of source indicated by ξ = {F, f} and the type of field indicated by η = {u, w} and the slownesses of the fast and slow compressional waves, s1, s2, and the shear wave, s3. Explicit expression of these three wave slownesses is given in Appendix A. The expressions for the amplitudes 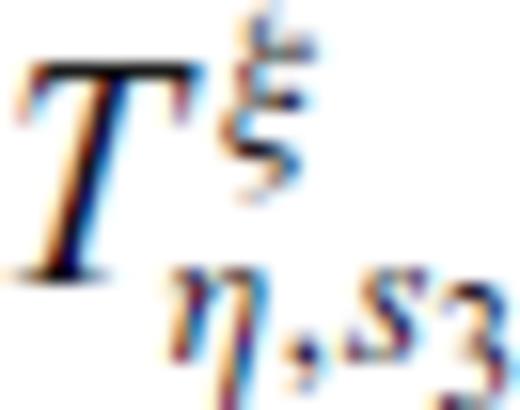 and
and  is given in Appendix B. Green's functions as defined in (14) and (15) can be easily reduced to the elastodynamic Green's function. In this case, only Green's function GF,u exists since in an elastic solid only the solid displacement field u due to a body force vector F0 is excited. Then, in eqs (15) one has to set the slow P-wave slowness to zero.
is given in Appendix B. Green's functions as defined in (14) and (15) can be easily reduced to the elastodynamic Green's function. In this case, only Green's function GF,u exists since in an elastic solid only the solid displacement field u due to a body force vector F0 is excited. Then, in eqs (15) one has to set the slow P-wave slowness to zero.Eqs (15) are equivalent to the fundamental solutions provided by Norris (1985). The solution from Norris (1985) was considered by Bonnet (1987) as overdetermined as a scalar source term is sufficient to describe sources in the fluid phase. Such a solution was derived by Boutin (1987) and is outlined in the next section.
3.2 Solid displacement and pore pressure representation
 and the relative displacement vector w from eqs (1), (2), (4) and (5) results into a vector wave equation for the solid displacement vector u and into a scalar wave equation for the pore-fluid pressure
and the relative displacement vector w from eqs (1), (2), (4) and (5) results into a vector wave equation for the solid displacement vector u and into a scalar wave equation for the pore-fluid pressure 

 are defined as
are defined as 




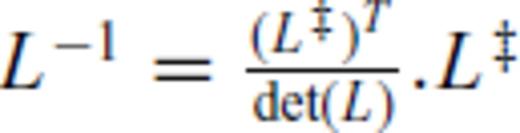 is the cofactor matrix of L and τ is the solution of the scalar equation
is the cofactor matrix of L and τ is the solution of the scalar equation 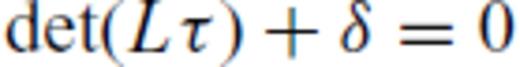 .
.
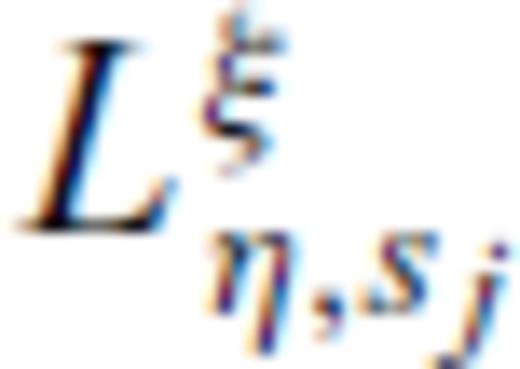 are given in the Appendix B. It is interesting to observe that the pressure field can be separated into a far-field term (first term proportional to 1/r) and a near-field (first term proportional to 1/r2). The scalar Green's function describing the pressure response p due to a volume source V is
are given in the Appendix B. It is interesting to observe that the pressure field can be separated into a far-field term (first term proportional to 1/r) and a near-field (first term proportional to 1/r2). The scalar Green's function describing the pressure response p due to a volume source V is 
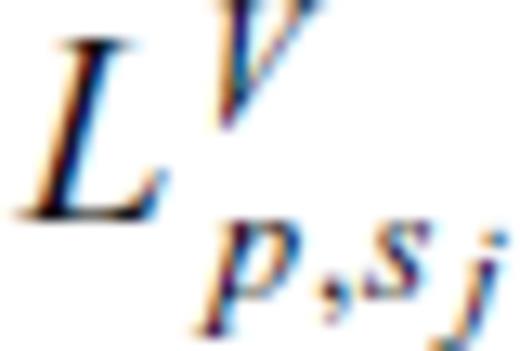 are listed in the Appendix B.
are listed in the Appendix B.Green's tensors (15), (23) and (24) fully describe a poroelastic medium as they provide solutions for all possible combinations of field variables (u, w, p) and for all possible forces (F, V). Note, however, that the single force in the fluid f is an unphysical source, as a fluid can only sustain hydrostatic pressure. This was already pointed out by Bonnet (1987).
Below we show that this inconsistency can be overcome by introducing the concept of moment tensor sources, thus replacing the single body force in the fluid phase by the body force equivalent of an explosive source.
4 Radiation Patterns
The radiation characteristics of waves emanating from various sources in poroelastic continua have only been investigated by Molotkov & Bakulin (1998). However, their work is restricted to the high-frequency range of Biot's theory. We generalize this work by analysing radiation characteristics in the full frequency range for relevant source configurations. A useful way to present the radiation characteristics of sources in elastic continua is presented by Chapman (2004). Here we follow this approach to construct radiation patterns of sources in poroelastic continua.

 ) multiplied by the corresponding amplitude
) multiplied by the corresponding amplitude 


4.1 Point force source
4.1.1 Far-field
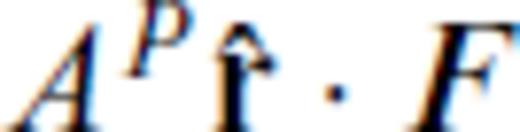 . Using spherical coordinates, the unit vector
. Using spherical coordinates, the unit vector  can be expressed as
can be expressed as 

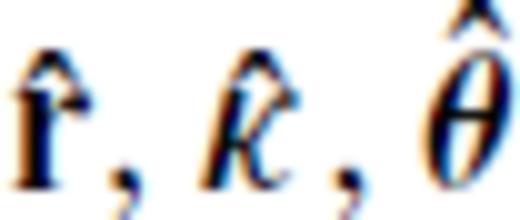 of the spherical coordinate system satisfy the identity
of the spherical coordinate system satisfy the identity 
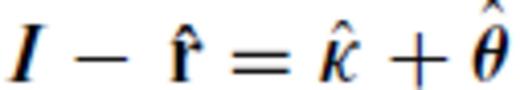 . The polarization of the S wave is in the plane perpendicular to the propagation direction
. The polarization of the S wave is in the plane perpendicular to the propagation direction  . If the z-axis is vertical, and the x−y plane is horizontal, it is common to introduce the notion of SH and SV waves. The horizontal component is
. If the z-axis is vertical, and the x−y plane is horizontal, it is common to introduce the notion of SH and SV waves. The horizontal component is 



Assuming a body force with z component only, i.e., F = (0, 0, Fz)T acting on a poroelastic solid, the radiation patterns of the solid displacement u and relative solid-fluid displacement are illustrated in Figs 1–3 using the frequency of 100 Hz and the material parameters as specified in Table 1. It can be observed that the shape of the radiation patterns is identical to the elastodynamic case. The P-wave displacement is largest in the direction of the applied force, whereas the S-wave displacement is largest perpendicular to the direction of the force vector. The amplitude of the S wave is larger than that of the P wave. In addition, the radiation pattern of the slow P wave has the same shape as that of the fast P wave, however its amplitude is significantly smaller (its amplitude has been amplified by a factor 108 to make it visible in Fig. 1).

Radiation pattern of solid displacement u due to a point force; the numbers 2 × 10−12 to 6 × 10−12 denote the displacement in meters along the radial axis; the radiation pattern of the slow P wave is enhanced by the factor 1 × 108.
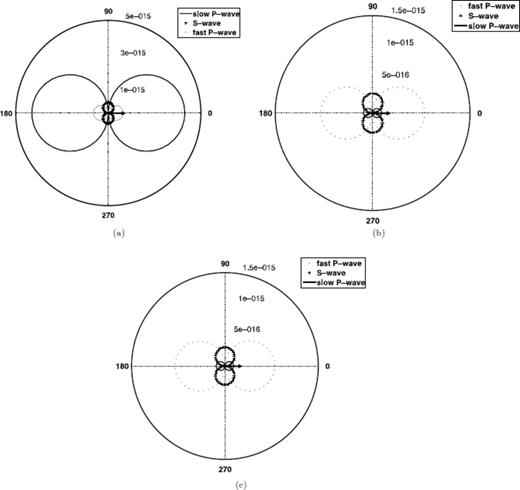
Radiation pattern of fluid displacement U due to single force for slow P wave, fast P wave and S wave, respectively; the numbers along the radial axis denote the displacement in meters; results are given for three different frequencies (a) 15 Hz, (b) 25 Hz, (c) 100 Hz.

Pressure field p due to a single force F for the slow and the fast P wave; the numbers 1.7504–7.6216 denote the pressure in Pascal along the radial axis; The slow P-wave dispersion is illustrated for three frequencies: 1 Hz, 10 Hz and 100 Hz.
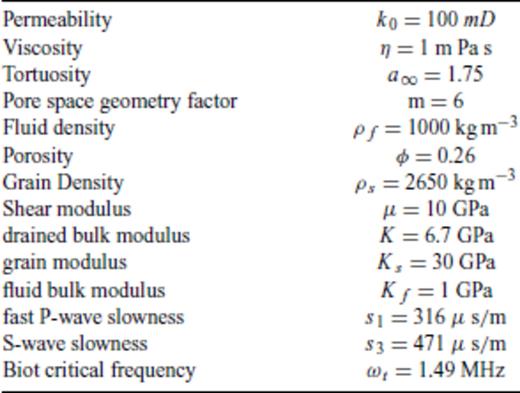
Material parameters of a typical sandstone used for the computations.
As a poroelastic medium is a two-phase system, the two P waves are differently manifested for different field variables. Comparing Figs 1 and 2(c) showing the solid and fluid displacement for 100 Hz, respectively a difference in magnitude of factor 4 from solid to fluid displacement can be observed. The slow P wave is easier to observe in terms of the fluid displacement especially for smaller frequencies (Figs 2a and b). Here the contribution of the fast P wave and S wave becomes significantly smaller than the slow P-wave displacement.
In Fig. 3, the pressure response p due to a single force F acting in z-direction (compare eq. 23) is computed for various frequencies. In this case only the fast and slow compressional waves exist. The values computed are plotted on a logarithmic scale. For low frequencies, the slow P wave (1-10 Hz) has a bigger amplitude than the fast P wave. For higher frequencies, the slow P-wave amplitude decays rapidly. An analogous case is the pressure field due to a volume source (eq. 24). The difference is that the slow P-wave amplitudes are larger while the fast P-wave amplitudes are smaller. The low-frequency limit of this case was studied by Chandler & Johnson (1981), Rudnicki (1986) and Müller (2006).
4.1.2 The near-field
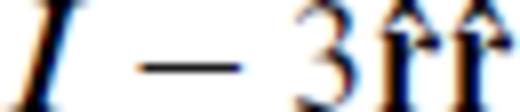 and the leading-order amplitude decays as 1/(ωsir2) (while the far-field decays with 1/r). Using again identity (31), the near-field polarization can be expressed as
and the leading-order amplitude decays as 1/(ωsir2) (while the far-field decays with 1/r). Using again identity (31), the near-field polarization can be expressed as 

The radiation pattern of the near-field is illustrated in Fig. 4. The form is the same for elastic and poroelastic media, only the amplitude differs. In source direction the amplitude is smaller than in perpendicular direction. This already indicates the difference in amplitude of P waves and S waves. Looking at eq. (23) it is obvious that the second term also decays like near-field terms but has the same polarization as the far-field. This means that for a pressure field due to a force vector (or displacement due to volume source) part of the energy decays with 1/r2 but with the same directivity as the far-field.
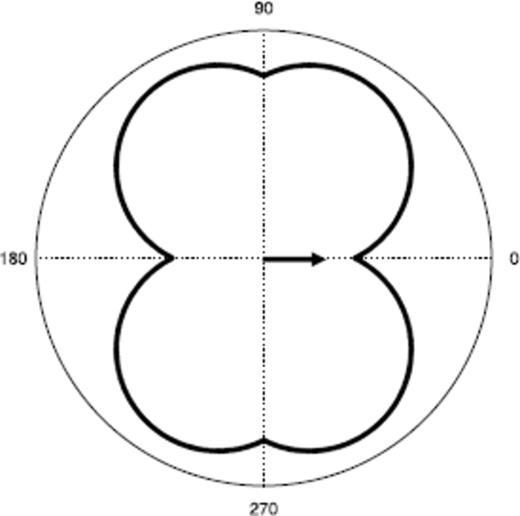
The radiation characteristics of the near-field. Only the geometry is illustrated without rock parameters.
4.2 Moment tensor sources


Body force equivalents of the moment tensor components (after Aki & Richards 2002).


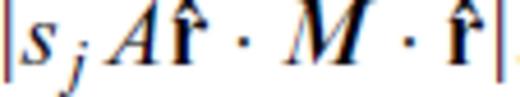 . Introducing spherical coordinates yields
. Introducing spherical coordinates yields 
 yielding
yielding 
 is using eq. (32)
is using eq. (32)
4.2.1 Monopole source



4.2.2 Dipole source




(colour online) Geometry of a 3-D radiation pattern for a dipole source acting on the solid phase; (a) P wave (b) S wave.

Dipole radiation pattern for solid displacement u; the numbers  denote the displacement in meters along the radial axis; Cross-section perpendicular to the x-y plane; the slow P-wave radiation pattern is amplified by factor 3 × 105.
denote the displacement in meters along the radial axis; Cross-section perpendicular to the x-y plane; the slow P-wave radiation pattern is amplified by factor 3 × 105.

Dipole radiation pattern for the relative displacement w; the numbers  denote the displacement in meters along the radial axis; Cross-section perpendicular to the x-y plane.
denote the displacement in meters along the radial axis; Cross-section perpendicular to the x-y plane.
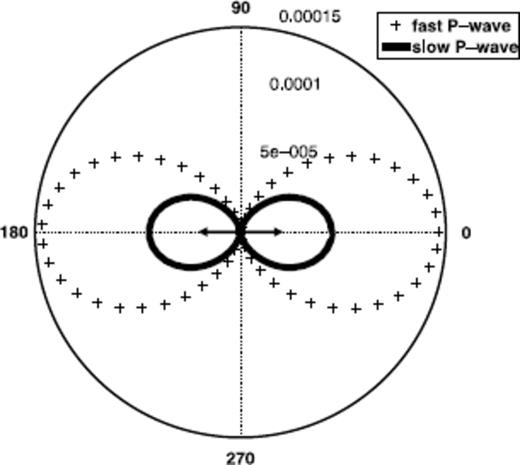
Dipole radiation pattern: pore pressure p; Cross-section perpendicular to the x-y plane; the numbers 5 × 10−5 to 0.00015 denote the pressure in Pascal along the radial axis; the slow P-wave displacement is amplified by factor 2 × 102; no shear wave is radiating in the fluid phase.
Figs 7–9 give a quantitative interpretation of a dipole source acting on a poroelastic medium. The responses for a medium described by the parameters in Table 1 are computed in terms of the solid displacement u, the relative displacement w and the pore pressure p. Similar to the single force case it can be observed that the solid displacement response is stronger by a factor of 5 compared to the relative displacement. The slow P wave is amplified by a factor of 3 ×105 for the solid displacement while it is not amplified for the relative displacement. Obviously, the slow P wave is stronger emphasized for w even though the overall magnitude is smaller. The strongest response for both P waves can be observed in the pressure field. The slow P wave is amplified by a factor of 3 × 105 but still has a significantly larger amplitude than for u and w.
4.2.3 Fluid dipole source


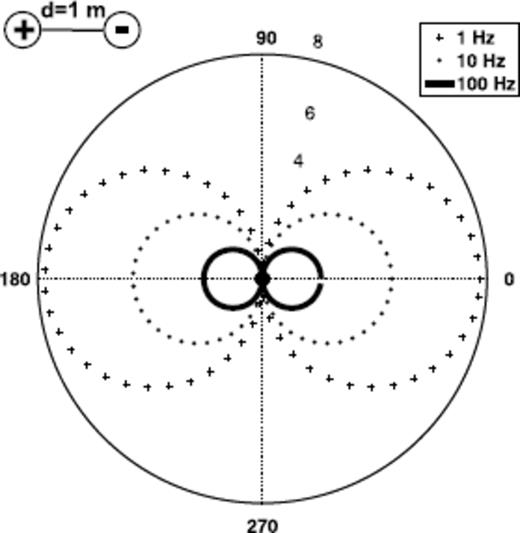
Fluid dipole radiation pattern: logarithm of the pore pressure p of the slow P wave for three frequencies; the numbers 4 − 8 denote the pressure in Pascal along the radial axis; point in the centre is the fast P wave that is negligibly small compared to the slow P wave.
4.2.4 Double-couple source


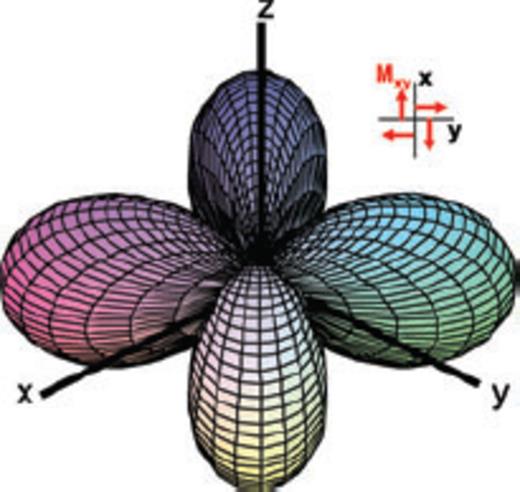
(colour online) 3-D P-wave radiation pattern of a double-couple source.



(colour online) 3-D radiation pattern of a double-couple source; (a) SH wave (b) SV wave.
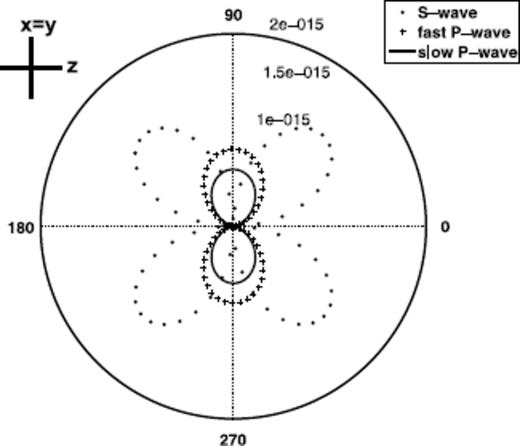
Double-couple radiation pattern: solid displacement u; the numbers 1 × 10−15 to 2 × 10−15 denote the displacement in meters along the radial axis; the slow P wave displacement is amplified by 5 × 105.
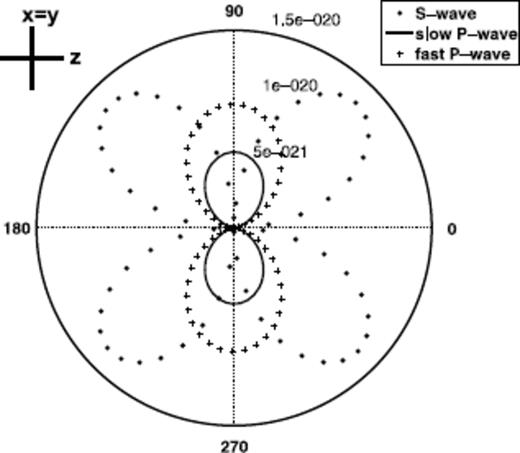
Double-couple radiation pattern: relative displacement w; the numbers 5 × 10−21 to 1.5 × 10−20 denote the displacement in meters along the radial axis.
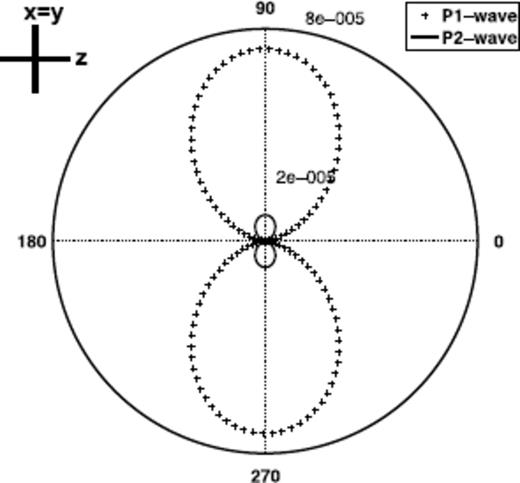
Double-couple radiation pattern: pore pressure p; the numbers 2 × 10−5 to 8 × 10−5 denote the pressure in Pascal along the radial axis; the slow P-wave radiation pattern is amplified by 1 × 105.
5 Conclusions
A review and comparison of different formulations of Green's functions for dynamic poroelasticity is provided. We conclude that previously reported sets of Green's functions can be used in a complementary sense such that all possible combinations of field variables and source types are included. We think that the unified presentation of Green's functions resolves the apparent contradictions between existing formulations. As expected, the shape of the radiation patterns of the fast compressional and shear wave is identical to their elastodynamic counterparts, however, complemented with the radiation pattern of Biot's slow wave. The relative magnitudes of the field variables shown in the radiation patterns can be very different for different source types. In particular, for any source acting in the fluid phase the pressure field is dominated by the Biot slow wave having compressional wave polarization. We introduce the concept of moment tensors for dynamic poroelasticity that allows us to overcome the inconsistency in Green's function formulation with respect to point force sources in the fluid phase.
Acknowledgments
We thank A. Bakulin (WesternGeco, Houston) who suggested to work on this topic at hand. The work of TMM was kindly supported by the Deutsche Forschungsgemeinschaft (contract MU1725/1-3).
References
Appendices
Appendix A: Biot Bulk Wave Slownesses


Appendix B: Amplitudes of Green's Functions

Author notes
CSIRO Petroleum.




