-
PDF
- Split View
-
Views
-
Cite
Cite
Alexander P Clark, Michael Clerx, Siyu Wei, Chon Lok Lei, Teun P de Boer, Gary R Mirams, David J Christini, Trine Krogh-Madsen, Leak current, even with gigaohm seals, can cause misinterpretation of stem cell-derived cardiomyocyte action potential recordings, EP Europace, Volume 25, Issue 9, September 2023, euad243, https://doi.org/10.1093/europace/euad243
Close - Share Icon Share
Abstract
Human-induced pluripotent stem cell-derived cardiomyocytes (iPSC-CMs) have become an essential tool to study arrhythmia mechanisms. Much of the foundational work on these cells, as well as the computational models built from the resultant data, has overlooked the contribution of seal–leak current on the immature and heterogeneous phenotype that has come to define these cells. The aim of this study is to understand the effect of seal–leak current on recordings of action potential (AP) morphology.
Action potentials were recorded in human iPSC-CMs using patch clamp and simulated using previously published mathematical models. Our in silico and in vitro studies demonstrate how seal–leak current depolarizes APs, substantially affecting their morphology, even with seal resistances (Rseal) above 1 GΩ. We show that compensation of this leak current is difficult due to challenges with obtaining accurate measures of Rseal during an experiment. Using simulation, we show that Rseal measures (i) change during an experiment, invalidating the use of pre-rupture values, and (ii) are polluted by the presence of transmembrane currents at every voltage. Finally, we posit that the background sodium current in baseline iPSC-CM models imitates the effects of seal–leak current and is increased to a level that masks the effects of seal–leak current on iPSC-CMs.
Based on these findings, we make recommendations to improve iPSC-CM AP data acquisition, interpretation, and model-building. Taking these recommendations into account will improve our understanding of iPSC-CM physiology and the descriptive ability of models built from such data.

Human-induced pluripotent stem cell-derived cardiomyocytes (iPSC-CMs) are an emerging tool in the study of cardiac arrhythmia mechanisms.
Their immature and heterogeneous action potential phenotype complicates the interpretation of experimental data and has slowed their acceptance in industry and academia.
We suggest that the leak current caused by imperfect pipette membrane seal during single-cell patch clamp experiments is partly responsible for causing this heterogeneity and the appearance of immaturity.
Using in vitro experiments and computational modelling, we show that this seal–leak current affects iPSC-CM action potential morphology, even under ‘ideal’ experimental conditions.
Based on these findings, we make recommendations that should be considered when interpreting, analysing and fitting iPSC-CM data.
Introduction
Human-induced pluripotent stem cell-derived cardiomyocytes (iPSC-CMs) are a renewable and cost-effective model for studying genetic disease mechanisms,1,2 drug cardiotoxicity,3 and inter-patient variability.4 Computational approaches have been developed to translate experimental results from iPSC-CMs to make predictions in adult cardiomyocytes.5 Such work attempts to bridge the critical gap that remains between the physiology of iPSC-CMs and excised adult human cardiac cells.
Whilst iPSC-CMs have transformed many areas of cardiac arrhythmia research, phenotypic heterogeneity and immaturity continue to stymie their potential impact.6,7 Investigating sources of these limitations and their biological implications is important as iPSC-CMs (and mechanistic models describing their behaviour) are used to inform increasingly complex clinical decisions.8,9 Studies of iPSC-CMs in a single-cell patch clamp context have indicated that their depolarized, highly varying resting membrane potential is primarily due to decreased inward rectifier potassium current (IK1) and increased funny current (If) compared with adult cardiomyocytes.10
Recently, findings from Horváth et al.11 and Van de Sande et al.12 indicate that the heterogeneous and depolarized resting membrane potential is also due, far more than previously thought, to a simple seal–leak current (Ileak). Relative to electrically coupled iPSC-CMs, they show a substantial depolarization in the resting membrane potential in isolated iPSC-CMs despite some cells having similar IK1 densities to human adult cardiomyocytes.11 These findings indicate that Ileak plays an important role in iPSC-CM AP morphology during single-cell patch clamp experiments.
Ileak is inversely proportional to the seal resistance (Rseal) formed between the micropipette tip and cell membrane during patch clamp experiments. A sufficiently large Rseal is expected to limit Ileak’s effect on AP morphology. Upon reviewing single-cell electrophysiological iPSC-CM studies, including those used to build iPSC-CM computational models,13–15 we found that studies do not report either an Rseal,10,16–19 a >1 GΩ Rseal acceptance criteria,20 or an average Rseal < 3 GΩ.11,12
In this study, through in vitro experiments and computational modelling, we show that Ileak affects iPSC-CM AP morphology, even above the Rseal values usually deemed acceptable in the literature. We show that Rseal cannot be easily compensated because it cannot be accurately measured during an experiment. Additionally, we posit that the background sodium current (IbNa) in iPSC-CM models may be overestimated and mimic the effects of leak on AP morphology. Ultimately, we argue that leak current should be considered when interpreting, analysing, and fitting iPSC-CM AP data.
Methods
Modelling Ileak
We added a leak equation to the Kernik13 and Paci14 iPSC-CM and ToR-ORd21 adult cardiomyocyte models. Knowing that leak acts as a depolarizing current in iPSC-CM studies and lacking information about specific charge carriers, we modelled Ileak as having a reversal potential of zero:22,23
where Rseal is the seal resistance and V denotes the membrane potential. The inverse of Rseal is the conductance, gseal. Note that more complicated equations for leak current (non-linear, and/or with a non-zero reversal potential) may be required in experiments where CaF2 seal enhancer is used.24
The effect of Ileak on the evolution of V was modelled as follows:
where Iion represents the sum of transmembrane currents and Cm is the membrane capacitance. Cm was set to 50 pF (the experimental average from the cells used in the present study) for the Kernik and Paci simulations, and for ToR-ORd, a value of 50 or 153 pF (the ToR-Ord baseline capacitance) was used unless specified otherwise.
Electrophysiological setup and data analysis
Perforated patch clamp experiments were conducted following a previously described protocol (see Supplementary Methods for more details).25
After contact was made with a cell and a seal of >300 MΩ was formed, the perforating agent slowly decreased the access resistance to the cell (usually 10–15 min). This low Rseal acceptance criterion was selected because we wanted to explore seal–leak effects above and below 1 GΩ. A series resistance (Rs) of 9–50 MΩ was maintained for all experiments. In this study, we used all cells from Clark et al.25 with membrane resistance (Rm) and Rs measurements acquired before and after current clamp recordings and that did not produce spontaneous alternans (n = 37 out of 40 cells). Rm, Cm, and Rs values were measured at 0 mV within 1 min prior to the acquisition of current clamp data.
All action potential (AP) features were calculated using a 10-s sample of current clamp data. The minimum potential (MP) was taken as the minimum voltage during this 10-s span. Maximum upstroke velocity (dV/dtmax), AP duration at 90% repolarization (APD90), and cycle length (CL) were averaged over all APs in the 10 s sample.
Rin as an estimate of Rseal
We calculate Rseal using a small test pulse in voltage clamp mode:26
Here, ΔVcmd is the applied voltage step, and ΔIout is the difference in recorded current from before to during the step. Once access is gained to a cell, it can be difficult to estimate Rseal, as the measured input resistance (Rin) depends on both Rm and Rseal [Eq. (4); Figure 1]. The effect of patch clamp series resistance on Rin measures was excluded from Eq. (4).
![Rseal cannot be measured directly once access is gained. Once access is gained, we can only measure the combined resistance Rin, which is equal to the parallel resistances of Rseal and Rm [Eq. (4)]. The presence of Rm introduces uncertainty when Rin is used to approximate Rseal, making it difficult to accurately correct for leak current effects. For simplicity, we have omitted other elements of this patch clamp diagram (e.g. series resistance and capacitance).](https://oup.silverchair-cdn.com/oup/backfile/Content_public/Journal/europace/25/9/10.1093_europace_euad243/5/m_euad243f1.jpeg?Expires=1750228087&Signature=HMiIm6I0Zu6htZFl~4gYYEbaxdSsjDtXsJ2blEsjRk6LYq~28Kr-Je5Tijb3NMz9Vsn3auG4d33bztA~AALPhdU112Ib3X8IAM7rZ0QsiB7EljdYS3D46eUqw2nt5UhW-hzZI~Fd5orOI1zHf8gIliGQqR3MXCZhAyzZBOhgB7BSMj-smzp-eqsOdxp5HrDJGcwSBOK2aYyKTFx0FZ6tzbZE9~TRVOA5sPO7-hkI-AaeTLgv94TPvcq3CFSegPO8xpPNUNy4E8SQgOJNFIWPIG46JQMOBYHnLd0TADYtoCas~8YIQWPqTvrkRjgauTm2PQZIhr9zPlNCYueyK5C1KQ__&Key-Pair-Id=APKAIE5G5CRDK6RD3PGA)
Rseal cannot be measured directly once access is gained. Once access is gained, we can only measure the combined resistance Rin, which is equal to the parallel resistances of Rseal and Rm [Eq. (4)]. The presence of Rm introduces uncertainty when Rin is used to approximate Rseal, making it difficult to accurately correct for leak current effects. For simplicity, we have omitted other elements of this patch clamp diagram (e.g. series resistance and capacitance).
The smallest Rseal considered was 300 MΩ, whilst Rs values ranged from 9 to 50 MΩ. An increase of Rs from 9 to 50 MΩ (a worst-case scenario we never observed) for a cell with a 300 MΩ Rseal would change Rin by 13%. So, whilst Rs can change in these experiments, it is unlikely to affect Rin by more than a few per cent, and Rseal is likely the predominant parameter affecting changes of Rin.
Additional methods
Additional methods can be found in the Supplementary material.
Results
Leak affects human-induced pluripotent stem cell-derived cardiomyocytes action potential morphology even at seal resistances above 1 GΩ
To investigate the effects of leak current on AP morphology, we simulated the addition of Ileak in the Kernik13 and Paci14 iPSC-CM models (Figure 2). Simulated AP recordings show that Ileak substantially alters AP morphology, even when Rseal ≥ 1 GΩ, a common threshold used in cardiac patch clamp experiments.20 For both models, decreases in Rseal depolarize the MP and cause a decrease in the dV/dtmax, likely due to an incomplete recovery of sodium channels at these depolarized MPs. Indeed, the Kernik model shows a transition to a small amplitude oscillation with very low upstroke velocity when Rseal < 3 GΩ and then depolarized quiescence when Rseal < 2 GΩ. Ileak effects on the APD90 differ for the two models—decreases to Rseal cause AP prolongation in the Paci model and AP shortening in the Kernik model. There are also differences in the effect of Rseal on CL: in the Kernik model, decreases in Rseal lead to a gradual decrease in CL, whilst in the Paci model, decreasing Rseal initially has limited effect on CL but then causes shortening as Rseal decreases below 5 GΩ.
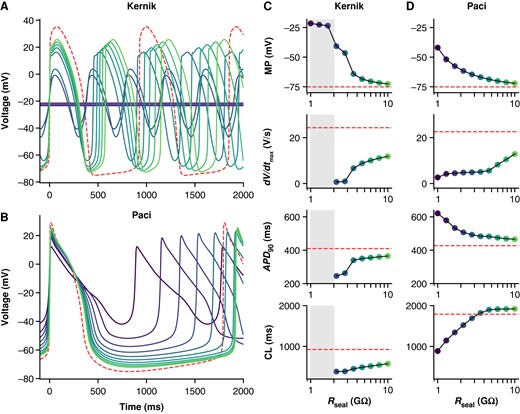
Effect of Rseal on Kernik and Paci APs. Simulations from the Kernik + leak (A) and Paci + leak (B) models, each with capacitance set to 50 pF (the experimental average), and Rseal set to values from 1 to 10 GΩ. The dashed (red) trace shows a baseline (leak-free) simulation. Four AP morphology metrics for the Kernik (C) and Paci (D) models are plotted against Rseal (displayed on log-scaled x-axis): MP, dV/dtmax, APD90, and CL. Grey boxes denote the Rseal values where the Kernik model is non-spontaneous. Abbreviations: APD90, action potential duration at 90% repolarization; CL, cycle length; dV/dtmax, maximum upstroke velocity.
Leak effects on adult cardiomyocyte action potentials are moderated by different current densities and increased ionic currents
The ToR-ORd adult cardiomyocyte model is also susceptible to Ileak effects, but the extent depends on cell capacitance (Figure 3). Simulations with Cm set to the average iPSC-CM capacitance (50 pF) result in substantial AP morphological changes when Rseal is between 1 and 2 GΩ. However, when Cm is set to a value in the range of adult human ventricular cardiomyocytes (153 pF), Ileak has little effect on AP morphology when Rseal is ≥1 GΩ (Figure 3B).
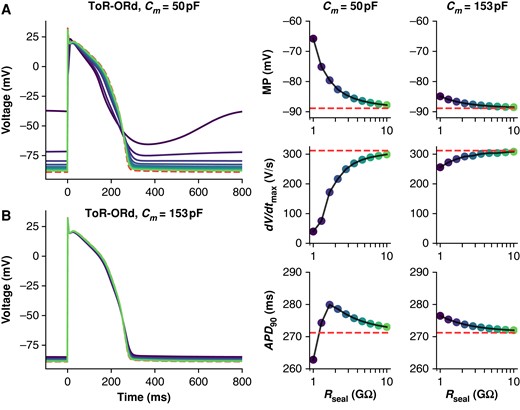
Effect of Rseal on ToR-ORd adult cardiomyocyte APs at 50 and 153 pF. Simulations from the ToR-ORd + leak model paced at 1 Hz with Cm set to 50 (A) and 153 pF (B), and Rseal set to values from 1 to 10 GΩ. The dashed (red) trace shows a baseline (leak-free) simulation. Three AP morphology metrics for the 50 and 153 pF models are plotted against Rseal (displayed on log-scaled x-axis): APD90, action potential duration at 90% repolarization; dV/dtmax, maximum upstroke velocity; MP, minimum potential.
Rseal is not stable
Unlike voltage clamp recordings, the effects of Ileak on AP morphology (measured in current clamp mode) cannot be corrected in post-processing. Current clamp leak compensation is a potential solution to the issue22,23 but requires an accurate measure of Rseal throughout the experiment.
Rseal cannot be accurately determined after access is gained because measures are contaminated by Rm; such resistance measures are a composite of these two resistances that we nominally refer to as Rin (see Figure 1 and Methods). It is, therefore, tempting to measure the value before gaining access and assume it remains unchanged for the duration of an experiment. To investigate this, we considered in vitro Rin measures taken two times during iPSC-CM experiments. Rin was measured with 5 mV steps from a holding potential of 0 mV (i.e. the leak reversal potential) before and after acquiring current clamp data. The data are skewed, with a mean of Rin = 2.71 GΩ and median of Rin = 0.82 GΩ.
The relative change in Rin from the first to the second time point was calculated and is plotted against the time elapsed between Rin measurements in Figure 4B. The median change of Rin is −15%. Because positive and negative changes cancel each other out in these statistics, we also inspected the absolute change, where we found a median of 20%. These data illustrate that Rin measurements often change over time. If we assume Rm is stable during experiments, this change in Rin should be attributed to Rseal and suggests that the average cell’s Rseal decreases (and therefore Ileak increases) over time.
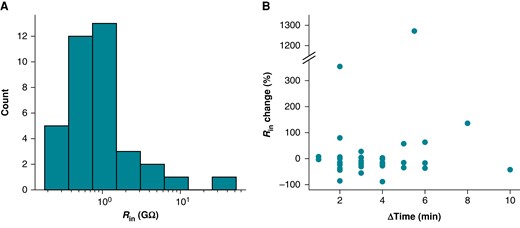
Rin changes during iPSC-CM experiments. (A) Distribution of initial Rin measurements from iPSC-CMs acquired with a +5 mV step from 0 mV. (B) The percentage change in Rin plotted against the time elapsed between Rin measurements. The interval between measurements ranged from 1 to 10 min. Time was recorded to the nearest minute, leading to the appearance of banding in the ΔTime measure.
Rin is not a good approximation of Rseal at any holding potential
A holding potential of −80 mV is a common choice for approximating Rseal with Rin measures. At this potential, sodium, calcium, and several potassium currents are expected to be largely inactive, but contributions from both IK1 and If must still be considered. Whilst IK1 is perhaps close to its reversal potential (and therefore small), If is not and can play a large role at this voltage.
We recently showed that If is present in at least some of the iPSC-CMs used in this study.25If is also present in both the Kernik and Paci models, and we found the dynamics of the Kernik If model to be quite similar to the in vitro data in this study (Figure 5A and B). Figure 5A shows an example cell’s response to an If-activating hyperpolarizing step before and after treatment with quinine, at a concentration expected to lead to 32% If block (these data are taken from a section of a larger protocol—see Clark et al.25Figure 6A). A change in total current of nearly 2 A/F is observed after holding at −120 mV for 1 s (Figure 5A). In Clark et al.,25 nine cells were treated with quinine, and the average change during the If-activating segment was 1.34 A/F. We found that these nine cells could be sorted into three triplets based on the amount of quinine-induced Iout change during the If segment: no/little sensitivity (ΔIout of 0–0.2 A/F), moderate sensitivity (Δ Iout of 0.7–1.2 A/F), and large sensitivity (Δ Iout of >1.9 A/F). Simulations using the Kernik model with 32% block of If show a change of 1 A/F (i.e. moderate change) in Iout (Figure 5B).

Ignoring the presence of If makes it impossible to accurately measure Rseal after gaining access. (A) Voltage clamp data acquired from an iPSC-CM before and after treatment with quinine, which is expected to block 32% of If at the concentration used. (B) Kernik model response at baseline and with 32% block of If. (C) Kernik + leak voltage clamp simulations conducted with Rseal = 1 GΩ, gK1 reduced by 90%, and gf set to 0 (solid line), 0.0435 (dotted line), or 0.087 nS/pF (dashed line). A voltage step from −80 to −75 mV was applied, as is commonly used to estimate Rin. This Rin value is sometimes used to approximate Rseal when the holding potential is near −80 mV. The amplifier-measured (Iout), total transmembrane (Iion), and leak currents (Ileak) are displayed. The Rin values calculated based on ΔIout are 2.03, 1.50, and 1.16 GΩ for the 0, 0.0435, and 0.087 nS/pF simulations, respectively. (D) Rin values are plotted against holding potential for Kernik + leak models with Rseal = 1 GΩ and gf equal to 0, 0.0435, or 0.087 nS/pF. The horizontal dotted line shows the true simulated Rseal value of 1 GΩ.
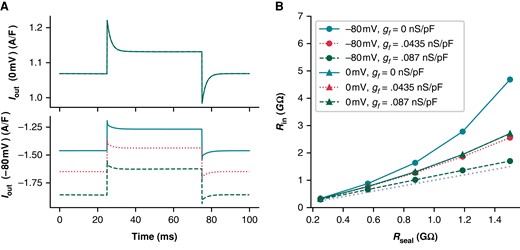
Rin predictions of Rseal are overestimated at the reversal potential for leak current. (A) The current response (Iout) for Kernik + leak models with a 1 GΩ seal and gf of 0 (solid line), 0.0435 (dotted line), or 0.087 nS/pF (dashed line) to a 50 ms + 5 mV voltage clamp step from 0 mV (top) or −80 mV (bottom). (B) Effect of Rseal on Rin measures for models with gf set to 0 (solid), 0.0435 (dotted), or 0.087 nS/pF (dashed). Rin was calculated with Eq. (3). The +5 mV voltage steps were taken from either 0 or −80 mV. The Rseal = Rin line (dotted, without symbols) is provided as a reference for when Rin correctly predicts Rseal. The 0 mV lines are overlapping, illustrating that Rin is not sensitive to gf at this voltage. The gf = 0.0875 nS/pF model at −80 mV provides the best estimate of Rseal.
To illustrate the effect of If on leak calculations, we compared simulations from Kernik + leak models with Rseal = 1 GΩ and with gf set to zero (i.e. not sensitive to quinine during hyperpolarizing step), the Kernik baseline value (gf = 0.0435 nS/pF, i.e. moderate sensitivity), or twice its baseline value (gf = 0.087 nS/pF, i.e. large sensitivity) (Figure 5C). We also reduced gK1 in these models to 10% of the baseline value to highlight the effects of If on Rin measures independent of IK1. The calculated Rin values for these models at −80 mV are 2.03 GΩ for gf = 0 nS/pF (little change), 1.50 GΩ for gf = 0.0435 nS/pF (moderate change), and 1.16 GΩ for gf = 0.087 nS/pF (large change) (Figure 5C). These simulations show that, at −80 mV, If contributes to Iout and affects measures of Ileak.
Using these same models, we then calculated Rin values at multiple holding potentials between −90 and +30 mV to determine whether we could find a potential where Rin is close to Rseal, thereby minimizing the prediction error (Figure 5D). The model predicts that 20 mV (Rin = 0.96 GΩ) minimizes the error in our approximation of Rseal. This does not mean that Rin measurements at 20 mV will always produce the best estimate of Rseal. Instead, it indicates the size of Iion does not change much when taking a 5 mV step from this potential. There is, however, a considerable amount of total current present, making this Rseal prediction sensitive to variations in the predominant ionic currents at this potential. Moreover, Ileak will be small and therefore more difficult to measure as 10 mV is close to the leak reversal potential (0 mV). It is also worth noting that the complex voltage- and time-dependent behaviour of transmembrane currents make Rin measures sensitive to both the duration and size of the voltage step (e.g. see supplement to Clerx et al.27). In summary, it is difficult to find a holding potential where Rseal can be measured without contamination from any transmembrane currents (i.e. where Ileak = Iout).
Taken together, these findings provide evidence to the claim that Rseal cannot be reliably measured in iPSC-CMs once access is gained.
Next, we compared the effect of If on Rm and investigated the error in assuming Rseal ≈ Rin, at both a 0 mV (i.e. Ileak reversal) and −80 mV holding potential. At 0 mV, the Kernik + leak model is not sensitive to changes in gf, as If is largely non-conductive (Figure 6A). However, due to an increased relative contribution of inward currents at 0 mV, the Kernik + leak model predicts a Rin with a large overestimation of Rseal (Figure 6B). This error increases as the true value of Rseal increases. Figure 6B also illustrates the sensitivity of the model to variations in gf at −80 mV, with Rseal estimation errors decreasing as gf increases; these errors also increase as Rseal increases. The improved prediction accuracy of the 0.087 nS/pF model at −80 mV is a coincidental side effect of doubling gf: with a different distribution of ion current densities or a larger baseline gf value, the same doubling could just as easily worsen Rseal predictions. For example, the Rin of an iPSC-CM with a large IK1 current may slightly underestimate Rseal at −80 mV—doubling gf in this case would result in a greater underestimation, increasing the error of the estimate.
Cm and Rin(0 mV) correlate with minimum potential
The iPSC-CMs used in this study displayed a heterogeneous phenotype (Figure 7), producing both spontaneously firing (n = 25) and non-firing (n = 12) current clamp recordings. Figure 7A shows three cells with very different baseline current clamp recordings: non-firing and depolarized (green), spontaneously firing with a short AP (teal), and spontaneously firing with a long AP (red). Non-firing cells (MP = −42 ± 8 mV) and cells with spontaneously firing APs were depolarized (MP = −54 ± 7 mV)—the spontaneously firing cells also had a shorter AP duration (APD90 = 128 ± 71 ms) (Figure 7B) relative to adult cardiomyocytes28 and iPSC-CM models.13,14
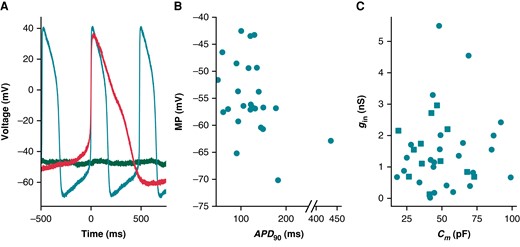
Cells appeared phenotypically heterogeneous, with uncorrelated variation in gin and Cm. (A) Current clamp recordings from three cells show phenotypic heterogeneity: non-spontaneous (green), spontaneous AP with short APD (teal), and spontaneous AP with long APD (red). (B) MP and APD90 for spontaneously beating cells (n = 25). Note the broken x-axis that allows us to display an outlying data point. (C) The relationship between Cm and gin for all cells (n = 37). Non-spontaneous cell data points are denoted with squares, whilst spontaneous are circles. APD90, action potential duration at 90% repolarization; MP, minimum potential.
We used linear regression analyses to determine if there is a correlation between gin/Cm and AP biomarkers. Here, we use gin (instead of Rin), as it reduces the spread of this variable and positively correlates with Ileak providing a more interpretable comparison with AP morphology. The values of each cell’s gin and Cm are shown in Figure 7C. Ileak’s effect on AP morphology is expected to scale directly with gin and inversely with Cm. This is because gin, even if a poor estimate, is expected to correlate with gseal (Figure 6B)
A given gleak will cause a smaller contribution in larger cells (i.e. cells with larger Cm), because the ionic currents are expected to scale with the size of the cell. For this reason, four AP biomarkers (MP, APD90, CL, and dV/dtmax) were compared with gin/Cm (Figure 8). The MPs of spontaneously firing (R = 0.44, P < 0.05) and non-firing (R = 0.76, P < 0.05) cells are positively correlated with gin/Cm (Figure 8A). This finding is in agreement with our in silico studies showing that increasing gseal, thereby increasing gin, will depolarize the cell (Figure 2). The other three biomarkers failed at least one of the assumptions required when conducting a linear regression analysis (see Supplementary Methods). There are no obvious trends when comparing gin/Cm with CL or dV/dtmax. The APD90 plot, however, indicates there may be some AP shortening as gin/Cm increases. Due to under-sampling and a lack of linearity, we cannot make any claims of significance between these two measures. Leak simulations with the models, though correlated, did not predict a linear relationship between gseal and these biomarkers (Figure 2C and D). However, the MP vs. gin/Cm relationship passes all tests of linear regression assumptions and trends in the same direction as the Kernik and Paci simulations in Figure 2.

Relationship between gin/Cm and AP biomarkers. (A) gin/Cm plotted against MP. Spontaneously firing cells are denoted as teal circles and non-firing cells as red squares. Linear regression fits to data from spontaneous (teal dashed, R = 0.47, P < 0.05), and non-firing (red dotted, R = 0.76, P < 0.05) cells are overlaid on the plot. No statistically significant relationship was found between gin/Cm and APD90 (B), CL (C), or dV/dtmax (D). APD90, action potential duration at 90% repolarization; CL, cycle length; dV/dtmax, maximum upstroke velocity; MP, minimum potential.
Fitting background currents in human-induced pluripotent stem cell-derived cardiomyocyte models can absorb and imitate Ileak
We used optimization to study the potential of linear background currents (e.g. sodium and calcium) to imitate leak effects (see Supplementary Methods). We fit the baseline Kernik model to a Kernik + leak model with Rseal = 5 GΩ (Figure 9), allowing only the background sodium (gbNa) and background calcium (gbCa) conductances to vary. These currents were selected because they were incorporated into the Kernik model without independent iPSC-CM experimentation or validation. The best-fit model had an increased gbNa (×7.0), whilst gbCa (×1.0) did not change much relative to the baseline model (Figure 9A). Whilst not a perfect match, the best-fit trace reproduced qualitative features of the baseline + leak trace, showing a depolarized MP and a smaller amplitude (Figure 9B). This indicates that increased IbNa can affect the AP in a fashion similar to Ileak such that mathematical iPSC-CM models may absorb Ileak effects by erroneously increasing background currents.
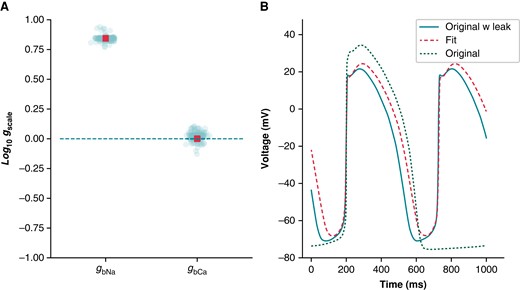
A simulated example of how leak can be absorbed into background currents: Kernik baseline model fit to Kernik + leak model. The IbNa and IbCa conductances (gbNa and gbCa) of the baseline Kernik model were fit to a Kernik + leak model (i.e. original + leak) with Rseal set to 5 GΩ using a genetic algorithm. (A) The conductances for all individuals (teal circles) and the best fit individual (red square) from the last generation. (B) Traces from the original baseline Kernik + leak model with a 5 GΩ seal (teal solid), the best fit model from the last generation (red dashed), and the original baseline Kernik model (green dotted).
Discussion
Leak current is a common and unavoidable experimental artefact that affects patch clamp recordings. In this study, using both model predictions and experimental data, we show that leak current: (i) affects iPSC-CM AP morphology, (ii) can vary during experiments, (iii) cannot be accurately estimated after access is gained to an iPSC-CM, and (iv) may be absorbed by linear equations for background currents when iPSC-CM models are fit to experimental AP data. During iPSC-CM current clamp studies, leak consideration often starts with a pre-rupture seal measurement (with a 1 GΩ threshold) and is ignored if the seal appears to remain stable throughout the study. Here, we argue leak effects should be quantitatively scrutinized during the acquisition, analysis, and fitting of experimental data. Furthermore, we believe cell-to-cell variation in seal resistance contributes to observed iPSC-CM AP heterogeneity—often attributed nearly entirely to variations in ionic current densities.
Leak affects action potential morphology
Simulations in chick embryonic cardiomyocytes, which are smaller than adult human cells (with model Cm = 25.5 pF), have previously shown that leak current substantially depolarizes the MP and shortens the CL, even with Rseal values of 5 GΩ.29 More recently, it was shown that in vitro iPSC-CMs were significantly depolarized during single-cell experiments, but not when cells were clustered.11,12 These results indicate that isolated iPSC-CMs are likely affected by leak current. Our in vitro and in silico findings support this conclusion and strengthen the argument that iPSC-CM AP morphology is strongly affected by leak current.
Our in silico work indicates that Ileak has a smaller effect on recordings of adult cardiomyocyte AP morphology when compared with iPSC-CMs (Figure 3B). This effect is strongly modulated by Cm, indicating the larger size of adult cardiomyocytes has a moderating effect on Ileak-induced AP changes. When the Ileak artefact in this adult model is normalized by the average iPSC-CM capacitance (50 pF, Figure 3A), Ileak substantially alters the AP shape at Rseal values above 1 GΩ. But the effects are much less than in the iPSC-CM model (Figure 2)—this indicates the ionic current expression profile of adult cardiomyocytes (e.g. greater IK1 and lower If density), in addition to cell size, and moderates the effects of Ileak on adult AP recordings. Thus, differentiation strategies that aim to mature the iPSC-CM phenotype (both in size and ionic current expression) will likely produce cells that are affected less by Ileak artefact.
Human-induced pluripotent stem cell-derived cardiomyocytes have long been defined by their immature and heterogeneous electrophysiological phenotype.10,30 Such features are due, at least in part, to the types of ion channels expressed and cell-to-cell variations in ionic current conductances.10,30 In this study, differences in the If responses to nine quinine-treated cells are an example of how iPSC-CM ionic currents can vary from one cell to the next. Heterogeneity in AP morphology and ionic current expression is also seen in primary adult cardiomyocytes.31–33
In this study, we show that Ileak also contributes to this immature and heterogeneous AP phenotype during single-cell patch clamp experiments. The relative importance of Ileak’s influence on AP shape varies amongst cells and depends on several factors, including Rseal, Cm, and the ionic current expression profile. Simulations indicate that the AP shape can be substantially altered (relative to non-patched cells), even when Rseal is equal to 10 GΩ, an unrealistically high acceptance criterion for iPSC-CM patch clamp studies. These factors, along with the potential for Rseal to change during an experiment, can confound drug and genetic mutation studies. For example, the irregular and depolarized phenotype (caused at least in part by Ileak) of iPSC-CMs in our recent cardiotoxicity study25 made it impossible to measure consistent cell-specific changes in spontaneous AP morphology from pre- to post-drug application.
The AP-altering effects of Ileak can be effectively eliminated by patching cells whilst in engineered heart tissue or monolayer. The electrical coupling of cells in these conditions results in an enormous effective capacitance, rendering Ileak an infinitesimal contributor to total current. Whilst this eliminates the Ileak artefact, it also comes at a cost—this approach does not allow for the direct measure of APs in individual cells, limiting the ability to study iPSC-CM heterogeneity. In addition, it is not possible to acquire voltage clamp data from cells in these conditions—as such, one could not acquire both AP and descriptive data about individual currents, as we recently have done in isolated cells.25
Predicting Rseal during experiments
Rseal can be well approximated prior to gaining access to a cell, but after perforation (or rupture), the presence of membrane currents makes it impossible to obtain an accurate measurement (Figure 5). Our in silico work shows that, even when currents such as If and IK1 are reduced to <10% of their baseline values, Rin (measured at −80 mV) is still a poor approximation of Rseal (Figure 6, solid black line).
To address these difficulties, we believe it may be feasible to use the pre-rupture Rseal and post-rupture Rin measures to calculate estimates of Rseal during an experiment. This approach would require an accurate measure of Rin just after access is gained. Using Rseal and the initial Rin, it is possible to calculate Rm (Figure 1). An estimate of Rseal could then be made at any time during the experiment, assuming the calculated Rm stays constant, by re-measuring Rin and using Eq. (4). This approach relies on two major assumptions: (i) the perforation/rupture step does not affect the seal, and (ii) a protocol or procedure exists that can be used prior to each measurement of Rin to ensure that the contribution of Rm is consistent. We cannot say for certain that these assumptions will always be valid. However, we believe that recording frequent Rin measurements, estimating Rseal, and scrutinizing changes are important steps for the correct interpretation of iPSC-CM current clamp data.
Correcting for Rseal during experiments
We believe these Rseal estimates should be used in a dynamic clamp leak compensation setup to address the limitations caused by a depolarized and variable MP. The approach works by injecting simulated currents into a cell in a real-time continuous loop during current clamp experiments.34IK1 dynamic clamp has been used on iPSC-CMs to attain quiescence at a MP below −70 mV so the cells can be paced at a desired frequency.25,35–37 A dynamically clamped leak compensation current has been implemented and used in manual patch clamp studies with neonatal mouse cardiomyocytes,22 demonstrating the potential of using such an approach with small cardiomyocytes. The effects of leak and the ability of leak compensation to recover adult cardiomyocyte behaviour have also been demonstrated in an in silico study.23 Together, these investigations demonstrate the potential of dynamic clamp as an experimental tool to simultaneously address shortcomings of the cells (i.e. IK1 density) and experimental setup (i.e. Ileak). This technique has the potential to improve the descriptive ability of iPSC-CMs when used in biophysical and drug investigations.
Inaccuracies in these estimates, however, will remain, resulting in the potential to under- or over-compensate. Over-compensation will hyperpolarize the MP and prolong Phases 1 and 2 of the AP, so we believe under-compensation is preferable. We suggest injecting a fraction of the full compensatory current to mitigate the risk of underestimating Rseal. The Nanion Dynamite8 sets the leak per cent compensation to 70%, which seems reasonable.38
Models of background currents can incorporate leak artefacts
The Kernik and Paci iPSC-CM models took ion-specific background currents from the ten Tusscher et al.39 model. These currents can trace their roots to the seminal work of Luo et al.,40 where they were included to help maintain physiologically realistic intra-cellular concentrations.
Direct measurements of IbCa and IbNa in iPSC-CMs have not been reported. The Kernik and Paci iPSC-CM models both adopted the ventricular39 formulation for IbCa and IbNa and then set the conductances of these currents by comparing model predictions of the AP with in vitro measurements in iPSC-CMs. We posit that IbNa is overestimated and compensates for the explicit consideration of leak current artefacts, a source of discrepancy between these models and reality. We expect consideration of leak when constructing iPSC-CM models to reduce background sodium current and result in a more realistic model of intact iPSC-CMs.
Modelling experimental artefacts
Whilst the effects of experimental artefacts in single-cell studies are well-established, consideration of them whilst building ion channel and AP models has been limited.41In silico studies investigating series resistance effects on voltage clamp recordings have been done in fast-activating currents, such as INa and Ito,42,43 but to our knowledge, artefact equations have not been included in the calibration process for widely used models of these currents—although the INa model by Ebihara et al.42 was incorporated directly into the widely copied INa model by Luo et al.40 Recently, Lei et al.44 demonstrated that coupling experimental artefact equations with an IKr mechanistic model improved predictions. These studies show that including experimental artefact equations in model fitting can improve the descriptive ability of the resulting electrophysiological models. As such, we believe experimental artefacts should be explicitly considered at the modelling phase and not ignored simply because a pre-determined minimum threshold is reached (e.g. 1 GΩ). Based on our findings, we believe cardiomyocyte models and especially iPSC-CM models should explicitly include leak currents when fitting to experimental current clamp data.
Recommendations
Our results provide important insights and recommendations for experimentalists and modellers alike:
Experimental: Rseal should be recorded before gaining access to a cell and Rin measured frequently during an experiment. It is important to measure Rin from a voltage that provides a consistent measure of Rm, such that any changes in Rin can be attributed to changes in Rseal.
Experimental: Dynamic injection of a leak compensation current can help a cell recover its native AP, including the MP. Because Rseal is difficult to measure during experiments and to avoid over-compensation, we advise under-compensation (e.g. 70%). Additionally, Rseal and Rin measures should be reported.
Modelling: Explicit inclusion of Ileak will improve the descriptive ability of iPSC-CM models. Whilst this may not always improve fits to AP data, it will take into account an important current affecting iPSC-CM recordings.
Limitations and future directions
This study has several limitations that should be considered during future investigations that may be affected by Ileak. First and foremost, when gathering these data for a previous study, we did not follow our new recommendation of recording the exact value of Rseal before gaining access and then measuring Rin just after perforation. Going forward, we hope to use these two values to predict Rseal at multiple time points during an experiment, as outlined in Section 3.2. Second, we only conducted these experiments in one cell line. Whilst our results appear similar to data from other labs,11 it would be useful to conduct this study on multiple cell lines in the same lab. Third, we did not attempt dynamic injection of a leak compensation current—in future work, we would like to investigate this as an approach to reducing cell-to-cell heterogeneity. Finally, the iPSC-CM models have innumerable differences from the cells used in this study, which is evident when comparing AP morphologies of in vitro cells (Figure 7A) to in silico models (Figure 2). However, the agreement that we did see between simulations and our in vitro data demonstrates the potential of improving the descriptive ability of iPSC-CM models by including a leak current.
Conclusion
In this study, we demonstrate that leak current affects iPSC-CM AP morphology, even at seal resistances above 1 GΩ, and contributes to the heterogeneity that characterizes these cells. Using both in vitro and in silico data, we showed the challenges of estimating Rseal after gaining access to a cell and that Rseal is subject to change during the course of an experiment. We also posit that background sodium current in iPSC-CM models may be responsible for masking leak effects in in vitro data. Based on these results, we make recommendations that should be considered by anyone who collects, analyses, or fits iPSC-CM AP data.
Supplementary material
Supplementary material is available at Europace online.
Acknowledgements
We thank the members of our labs for their support and feedback at every step of the way.
Funding
This work was supported by the National Institutes of Health (NIH) National Heart, Lung, and Blood Institute (NHLBI) grants U01HL136297 to D.J.C. and F31HL154655 to A.P.C., the Wellcome Trust via a Senior Research Fellowship to G.R.M. (grant number 212203/Z/18/Z), Universidade de Macau via a UM Macao Fellowship and support from FDCT Macao (Science and Technology Development Fund, Macao S.A.R. (FDCT) reference number 0048/2022/A) to C.L.L., and the MKMD programme of the Netherlands Organization for Health Research and Development (grant number 114022502) to T.P.B.
Data availability
All data, code, and models can be accessed from GitHub (https://github.com/Christini-Lab/iPSC-leak-artifact).
Translational perspective
Human iPSC-CMs have emerged as a promising translational tool to study human cardiac physiology outside of the clinic. They have been particularly useful to investigate cell-level pro-arrhythmic substrates, including genetic mutations and ion channel-blocking drugs, and play a critical role as a model for validating drug effects on human whole-cell electrophysiology in the Comprehensive in vitro Proarrhythmia Assay (CiPA) initiative. However, the depth of insights from iPSC-CM data is often limited by inter- and intra-lab heterogeneity caused, at least in part, by patch clamp experimental artefact. In this manuscript, we show how the seal–leak current is an often-overlooked artefact that confounds studies with iPSC-CMs. Ultimately, the findings and recommendations within this manuscript will improve the use of iPSC-CMs as an in vitro model to study cardiac electrophysiological diseases and patient-specific treatment strategies.
References
Author notes
Conflict of interest: T.P.B. is an Editorial Consultant of EP Europace and was not involved in the peer review process or publication decision. All remaining authors have declared no conflicts of interest.



