1-20 of 7081
Image
Numerical errors under different norms of the FEM ( 5.2 ) at : (a) Case ... Get access
Published: 30 April 2025
Numerical errors under different norms of the FEM ( 5.2 ) at : (a) Case 1; (b) Case 2; (c) Case 3.
Image
Snapshots of the curve evolution using the FDM (first row), FEM (second row... Get access
Published: 30 April 2025
Snapshots of the curve evolution using the FDM (first row), FEM (second row) and FEM-TM (third row) with for Case 3. The parameters are chosen as and .
Image
Evolution of the geometric quantities using the FDM, FEM and FEM-TM with ... Get access
Published: 30 April 2025
Evolution of the geometric quantities using the FDM, FEM and FEM-TM with for Cases 1–3 is illustrated in the first through third rows, respectively. (a) Perimeter ; (b) Relative area loss ; (c) Mesh ratio function . The parameters are chosen as and .
Image
Numerical errors under different norms for the FDM ( 5.1 ) at : (a) Case... Get access
Published: 30 April 2025
Numerical errors under different norms for the FDM ( 5.1 ) at : (a) Case 1; (b) Case 2; (c) Case 3.
Image
Numerical errors under different norms of the FEM-TM ( 5.4 ) at : (a) Ca... Get access
Published: 30 April 2025
Numerical errors under different norms of the FEM-TM ( 5.4 ) at : (a) Case 1 with ; (b) Case 1 with ; (c) Case 2 with ; (d) Case 3 with .
Image
Snapshots of the curve evolution using the FDM (first row), FEM (second row... Get access
Published: 30 April 2025
Snapshots of the curve evolution using the FDM (first row), FEM (second row) and FEM-TM (third row) with for Case 2. The parameters are chosen as and .
Image
Evolution of geometric quantities using the FEM-TM with different for C... Get access
Published: 30 April 2025
Evolution of geometric quantities using the FEM-TM with different for Case 1. (a) Perimeter ; (b) Relative area loss ; (c) Mesh ratio function . The parameters are chosen as and .
Journal Article
Convergence analysis of three semidiscrete numerical schemes for nonlocal geometric flows including perimeter terms Get access
IMA Journal of Numerical Analysis, draf015, https://doi.org/10.1093/imanum/draf015
Published: 30 April 2025
Image
Illustration of (a) the definition of and , (b) the comparison of th... Get access
Published: 30 April 2025
Illustration of (a) the definition of and , (b) the comparison of the projection distance and the function -norm .
Image
Snapshots of the curve evolution using the FDM (first row), FEM (second row... Get access
Published: 30 April 2025
Snapshots of the curve evolution using the FDM (first row), FEM (second row) and FEM-TM (third row) with for Case 1. The parameters are chosen as and .
Image
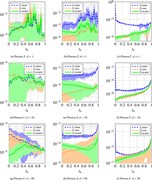
Mean loss and MSE values of the process from DBDP, OSM and DLBDP scheme... Get access
Published: 28 April 2025
Mean loss and MSE values of the process from DBDP, OSM and DLBDP schemes at discrete time points using the validation sample in Example 1 , for and . The STD of the loss and MSE values is given in the shaded area.
Journal Article
A backward differential deep learning-based algorithm for solving high-dimensional nonlinear backward stochastic differential equations Get access
IMA Journal of Numerical Analysis, draf022, https://doi.org/10.1093/imanum/draf022
Published: 28 April 2025
Image
Mean MSE values of the processes from DBDP, OSM and DLBDP schemes over ... Get access
Published: 28 April 2025
Mean MSE values of the processes from DBDP, OSM and DLBDP schemes over the discrete time points using the testing sample in Example 1 , for and . The STD of MSE values is given in the shaded area.
Image
Mean MSE values of the processes from DBDP, OSM and DLBDP schemes over ... Get access
Published: 28 April 2025
Mean MSE values of the processes from DBDP, OSM and DLBDP schemes over the discrete time points using the testing sample in Example 3 , for and . The STD of MSE values is given in the shaded area.
Image
Mean MSE values of the processes from DBDP and DLBDP schemes over the d... Get access
Published: 28 April 2025
Mean MSE values of the processes from DBDP and DLBDP schemes over the discrete time points using the testing sample in Example 4 , for and . The STD of MSE values is given in the shaded area.
Journal Article
Spatio-temporal Lie–Poisson discretization for incompressible magnetohydrodynamics on the sphere
IMA Journal of Numerical Analysis, draf024, https://doi.org/10.1093/imanum/draf024
Published: 26 April 2025
Journal Article
On the approximation of singular functions by series of noninteger powers
IMA Journal of Numerical Analysis, draf006, https://doi.org/10.1093/imanum/draf006
Published: 26 April 2025
Image
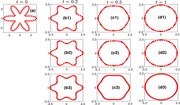
Phase portrait for (left) and (right) for the Kirchhoff system, Kir...
Published: 26 April 2025
Phase portrait for (left) and (right) for the Kirchhoff system, Kirchhoff integrable case. Component is shown against , and component is shown against .
Image
Phase portrait for (left) and (right) for the Kirchhoff system, Lya...
Published: 26 April 2025
Phase portrait for (left) and (right) for the Kirchhoff system, Lyapunov–Steklov–Kolosov integrable case. Component is shown against , and component is shown against .
Image
Variation of the smallest eigenvalue of for Hazeltine’s equations. The ...
Published: 26 April 2025
Variation of the smallest eigenvalue of for Hazeltine’s equations. The order of the magnitude variation indicates the exact preservation of the spectrum.
